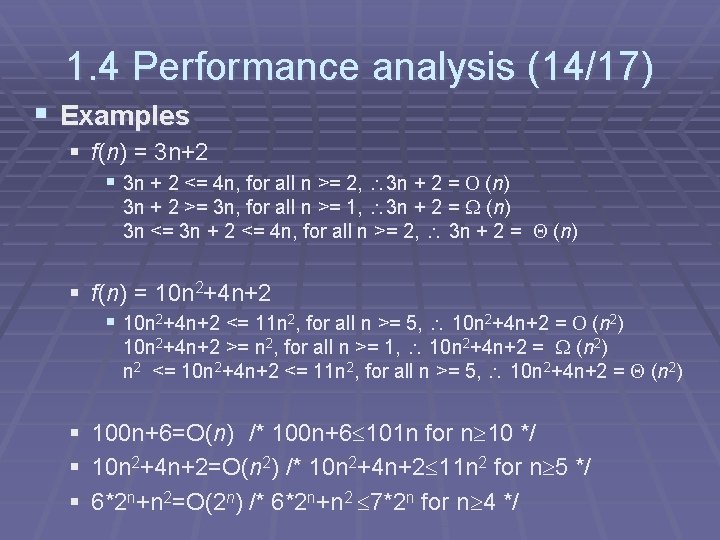
N24n = 1 Now the clever bit Take the coefficient of n , which is 4 , divide by two, giving 2 , and finally square it giving 4 Add 4 to both sides of the equation On the right hand side we have 1 4 or, (1/1) (4/1) The common denominator of the two fractions is 1 Adding (1/1) (4/1) gives 5/1 $\begingroup$ A lot of it is just keeping really good account of what is assumed in the inductive step and what is to be provedHere you can see that we can assume the sum of the numbers up through $3n2$ is $\frac{n(3n1)}{2}$, and this fact is used in the very first equationCalculadoras gratuitas passo a passo para álgebra, trigonometria e cálculo

2 3 4n 1 2n 1 N 3 N 3 4 3find The Value Of N Brainly In
How many solutions does 2(n-1)+4n=2(3n-1)
How many solutions does 2(n-1)+4n=2(3n-1)-Where we use a similar proof to that of Theorem 97(c) to show (3n)1=(4n2)!1 Thus the radius ofYes, 2 equals 2 Therefore, the answer is infinitely many solutions, meaning that n can be any real number
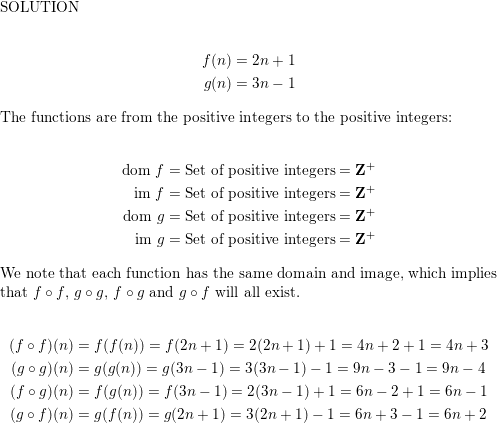



Let F And G Be Function From The Positive Integers To The Positive Integers Defined By The Equations F N 2n 1 G N 3n 1 Find The Compositions F Math Circ Math F G Math Circ Math G F Math Circ Math G And G Math Circ Math F Homework
Simple and best practice solution for (3n2)(4n1)=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so 6 Answers6 As a hint you apply ln to both sides of limit Per the hint in comments, we squeeze the limit expression between ( 3 n) 1 / n and ( 4 n) 1 / n, both of which evaluate to 1, so the limit is 1 (It's easier to show that lim n → ∞ a 1 / n = 1 for a ≥ 1 )A The range is the set of all real numbers
Click here👆to get an answer to your question ️ n→∞ n(2n 1)^2/(n 2)(n^2 3n 1) is equal to Please see below Induction method is used to prove a statement Most commonly, it is used to prove a statement, involving, say n where n represents the set of all natural numbers Induction method involves two steps, One, that the statement is true for n=1 and say n=2 Two, we assume that it is true for n=k and prove that if it is true for n=k, then it is also true for n=k1 First1Which of the following shows the best next step to prove the following by mathematical induction?
2 Answers 0 votes quantity n times six n squared minus three n minus one all divide by two Mathematical induction is a special way of proving things It has only two steps Step 1) Statement is true for n = 1 Step 2) one part being assume the statement is true for n = k prove the statement is true for " k 1" 2 (n 1) 4n = 2 (3n 1) Let's simplify this to solve it 2n 4n 2 = 6n 2 Rearrange the left side of the equation 6n 2 = 6n 2 Add 2n 4n 6n 6n Subtract 6n from both sides 2 = 2?Experts are tested by Chegg as specialists in their subject area We review their content and use your feedback to keep the quality high



In Exercises 5 18 Use Mathematical Induction To Chegg Com



1 0 7 Points Choose All Appropriate Answers The Chegg Com
First we find the common difference (CD) between first two terms of series CD = 4n 1 (n 2) CD = 4n 1 n 2 CD = 3n 1 Again find the common difference between 4n 1 and 5n 2 as CD = 5n 2 (4n 1) CD = 5n 2 4n 1 CD = n 3 I3n 1 2n 5 3 2 = 13 2(2n 5) 13 2(2N 5) < ; 2(n1) 4n= 2 (3n 1) Answers 3 Get Other questions on the subject Mathematics Mathematics, 1500, 6clevare If 1 is divided by the sum of y& 4 the result is equal to 3 divided by 4 find the value of m?



2




Prove The Following By Using The Principle Of Mathematical Induction For All N N 1 3 3 5 5 7 2n 1 2n 1 N 4n 2 6n 1 3 Mathematics Shaalaa Com
( I wrongly noted the question Instead of writing 3r/n i wrote r/n, but the solution will be similar to below one(Sorry) This is very interesting question a lot of students get confused in these type of problems How they approach, they first tClick here👆to get an answer to your question ️ If numbers n 2 , 4n 1 and 5n 2 are in AP, find the value of n and its next two termsThere are several proofs by



Http Www Math Ualberta Ca Isaac Math222 W07 Soln3 Pdf




1 Let An 2n 3n 1 A Determine Whether An Chegg Com
N=1 1 n1/2 Solution The given series is a pseries P 1 np with p = 1 2 We know that a pseries converges only if p > 1, so this series diverges Let's use the Limit Comparison test to show this The series P 1 n diverges and lim n→∞ 1 n1/2 1 n = lim n→∞ n n /2 = lim n→∞ n1/2 = ∞ Therefore, by Part (iii) of the Limit Comparison3^n>n*2^n, n≥1 1When n=1, the formula is valid because 3^1 1*2^1 3>2 2Assuming that 3^k>k*2^kWe can use the root test to compute limsupja kj 1=k = limja 2n1j 1=(2n1) = lim 3n p n 1=(2n1) = lim 3n=(2n1) n1=(4n2) = lim31=2(3n) 1=(4n2) = 31=2;




Evaluate Each Of The Following Expressions For N 2 Gauthmath




Dtaa Center Redundancy N 1 N 2 Vs 2n Vs 2n 1 Part Ii
Assume n>=1, now count the terms and treat the 2n's and the odd numbers separately Reply Likes 1 person #3 1MileCrash 1,331 40 No, subtracting the 2(n1) from the series for 3n² does not get you the series for 3(n1)² because these series are not infinite Think about it, how could I subtract something from 3n² and get aSˇc´ıtajte nasleduju´ce rady, ak konverguju´ alebo dok´aˇzte, ˇze diverguju´ X1 n=2 3n1 6·22n3, X1 n=1 1 4n2 1, X1 n=1 n2 n! Use Mathematical Induction to show that the statement 2 6 10 (4n – 2) = 2n^2 is true precalculus Can you check my answers?



Ex 9 4 10 Find Sum Of Series Nth Terms Is 2n 1 2 Ex 9 4
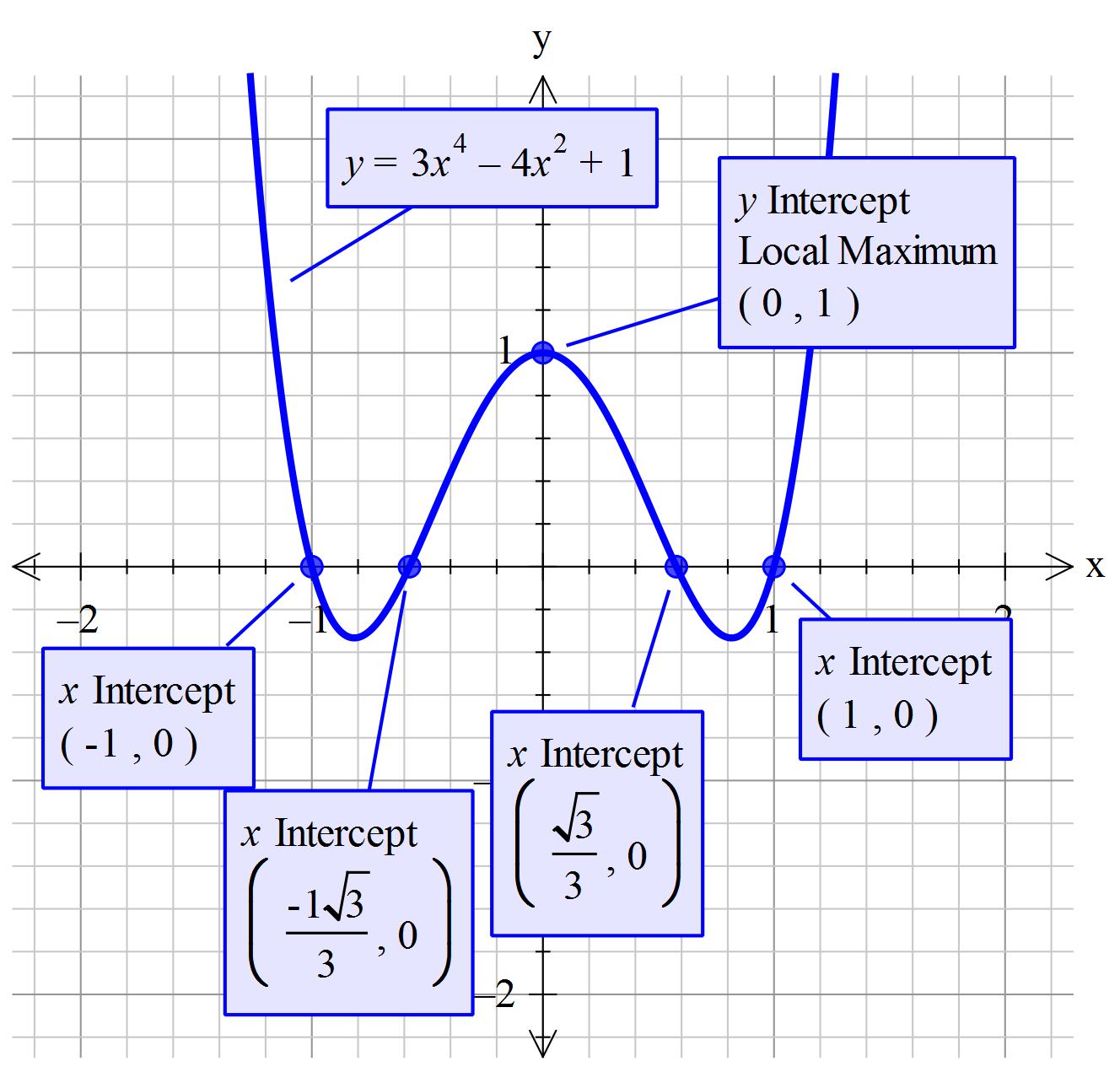



How Do You Solve 3n 4 4n 2 1 Socratic
N = 2 3 n = 2 3 Set the next factor equal to 0 0 and solve Tap for more steps Set the next factor equal to 0 0 4 n 1 = 0 4 n 1 = 0 Subtract 1 1 from both sides of the equation 4 n = − 1 4 n = 1 Divide each term by 4 4 and simplify2 (n1)4n=2 (3n1) Simple and best practice solution for 2 (n1)4n=2 (3n1) equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itIs the series ∞∑n=1 ((−2)^(3n)n^n)/((3n 1)^n) conditionally convergent, absolutely convergent, or divergent Expert Answer Who are the experts?



Sequences




Which Expression Is Equivalent To 2 3n 2 9n A 16n B 3n 8 C 4 3n 1 D 4 3n 4 Brainly Com
N=1 2n 3n 4n Answer Rewriting slightly, the given series is equal to X1 n=1 2 n 4n 3n 4n = X1 n=1 2 4n X1 n=1 3n 4n Since both of these series are convergent geometric series, I know the original series converges, so it remains only to determine the sum Notice that X1 n=1 2n 4n = 2 4 4 16 8 64 = 2 4 1 2 4 4 16 = X1(3n 1) (3n 1) (3n 1) (3n 1) (3n 1) (3n 1) = 2x The above line being obtained by adding the two equations together Note that we have that where there are exactly n copies of (3n 1) in the sum Thus n(3n 1) = 2x, so x = n(3n 1) 2 Next we prove by mathematical induction that for all natural numbers n, 1 4 7 (3n 2) = nWatch Video in App This browser does not support the video element 376 k 19 k Answer Step by step solution by experts to help you in




Prove The Following 1 4 7 3n 2 N 3n 1 2 Homeworklib



Solution Perform The Operations And Simplify 3n 2 5n 2 12n 2 13n 3 N 2 3n 2 4n 2 5n 6
27 Multiply (n1)2 by (n1) The rule says To multiply exponential expressions which have the same base, add up their exponents In our case, the common base is (n1) and the exponents are 2 and 1 , as (n1) is the same number as (n1)1 The product is therefore, (n1)(21) = (n1)3 The value of lim_(n to oo) (2n^(2) 3n 1)/(5n^(2) 4n 2) equals Updated On To keep watching this video solution for FREE, Download our App Join the 2 Crores Student community now! 2 ( n1 ) 4n= 2( 3n1 ) Distribute 2n 2 4n = 6n 2 Combine like terms 6n 2 = 6n 2 Subtract 6n from each side 6n26n = 6n26n2 = 2 This is always true so there are infinite solutions thank you New questions in Mathematics Based on the graph, which statement about the function is true?




Exercises Teaching Mathematics




Icsepapers Evaluate A 2n 1 A 2n 1 2n 1 A N 4n 1 A 2 2n 3
Which veri es the limit (You may also use 13 2(2n5) 4 n to choose an easier N) (2)(8 points) Let x n y n z n for all n2N If lim(x n) = lim(z n) = l, show that lim(y n) exists and equals l Proof Given >0;since (x n) !l, 9N 1 2N such that l #sum_(n=1)^oo 1/((3n2)(3n1))# There is an infinity sign on the top of the summation sign and n=1 on the bottom Please write in details;Answers 1 continue Mathematics, 1730,



What Is Program A Set Of Instructions Data



1
find the inverse laplace transform of L^1(s^23s4/s^3) A's present age is 4 yr more than B's age after6 yr The sum of the present age of A and Btogether is 70 yrSigma(n=1, infinity) (1)^(n1)/(4n^2 1)Test the series for convergence or divergence4n2=6(1/3n2/3) One solution was found n = 3 Rearrange Rearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation




2 3 4n 1 2n 1 N 3 N 3 4 3find The Value Of N Brainly In
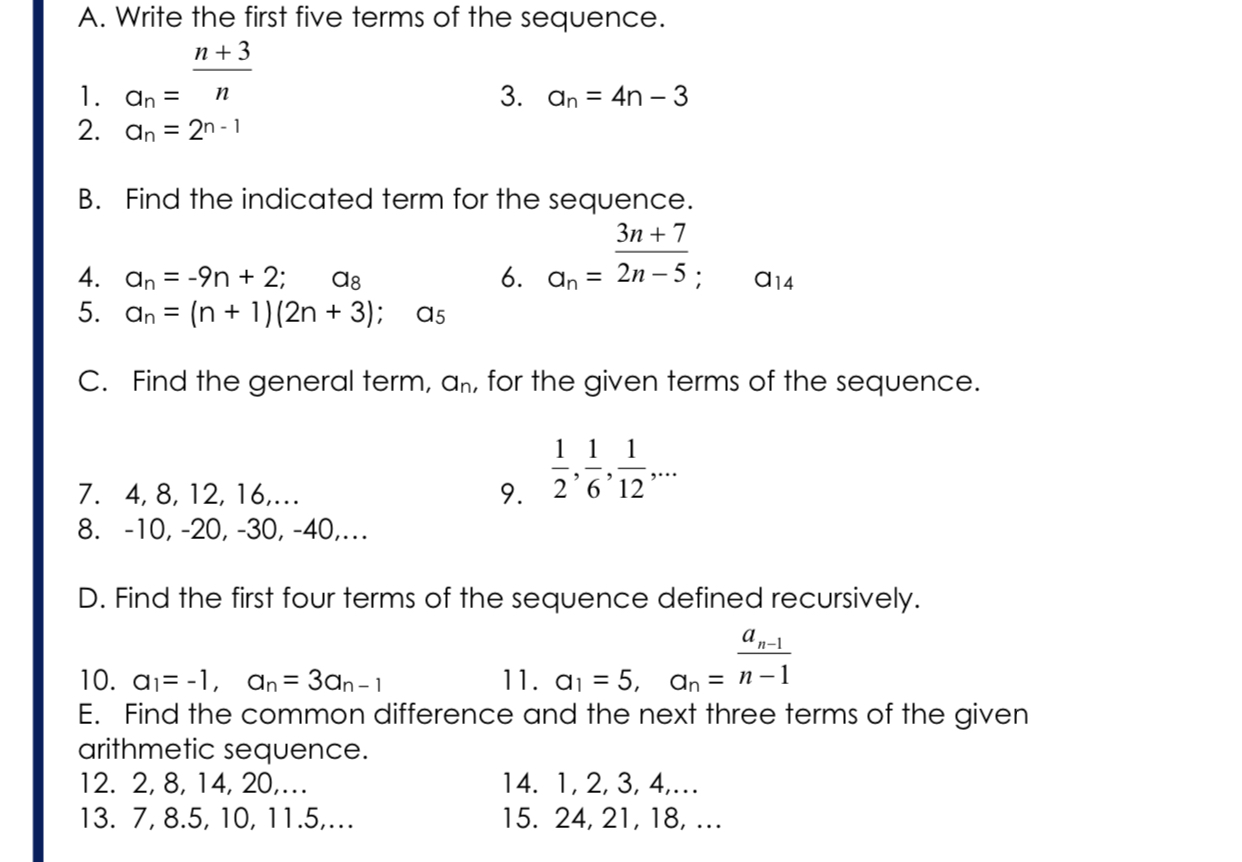



Answered A Write The First Five Terms Of The Bartleby
4 P 1 n=1 n2 41 Answer Let a n = n2=(n4 1) Since n4 1 >n4, we have 1 n41 < 1 n4, so a n = n 2 n4 1 n n4 1 n2 therefore 0 What is the answer for 2(n1)4n=2(3n1) Answers 3 Get Other questions on the subject Mathematics Mathematics, 1330, 22justinmcminn Which equation represents a proportional relationship that has a constant of proportionality equal to 1/5 Answers 1Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



The Sum Of N 2n 3n Terms Of An Ap Are S1 S2 S3 Respectively Prove That S3 3 S2 S1 Arithmetic Progressions Maths Class 10



1
In an induction proof of the statement 4710(3n1)=n(3n5)/2 the first step is to show that the statement is true for some integers n Note3(1)1=13(1)5/2 is true Select the steps required to complete the proof A)Show that the statement is true for any real number k Show that the statement is true for k1 B)Assume that the statement is true for some positiveSolve for n 2 (n1)4n=2 (3n1) 2(n − 1) 4n = 2(3n − 1) 2 ( n 1) 4 n = 2 ( 3 n 1) Simplify the left side Tap for more steps Simplify each term Tap for more steps Apply the distributive property 2 n 2 ⋅ − 1 4 n = 2 ( 3 n − 1) 2 n 2 ⋅ 1 4 n = 2 ( 3 n 1) Multiply 2 2 by − 1 1 These configurations take various forms, such as N, N1, N2, 2N, 2N1, 2N2, 3N/2, among others These multiple levels of redundancy topologies are described as NModular Redundancy (NMR) N refers to the bare minimum number of independent components required to successfully perform the intended operation
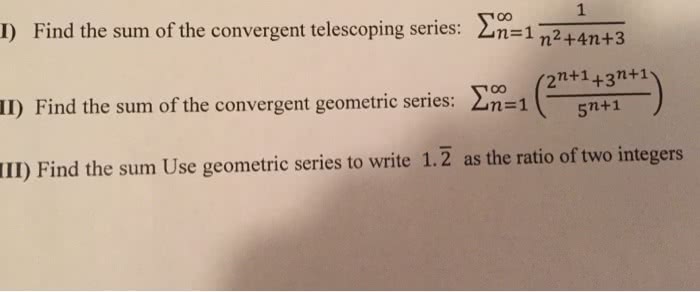



Oneclass 1 Find The Sum Of The Convergent Telescoping Series 2n 1 4n 3 11 Find The Sum Of The Co



What Is The Value Of Lim N Infinity N N N 3n 3 4n 2 1 Quora
Factor 12n^{3}4n^{2}3n1 he Related Symbolab blog posts Practice Makes Perfect Learning math takes practice, lots of practice Just like running, it takes practice andDetermine whether the series is absolutely convergent, conditionally convergent, or divergent fullscreen help_outline Image Transcription close 4n 3n 1 2n 3 16 n=1 fullscreen2 k odd 0 k even;
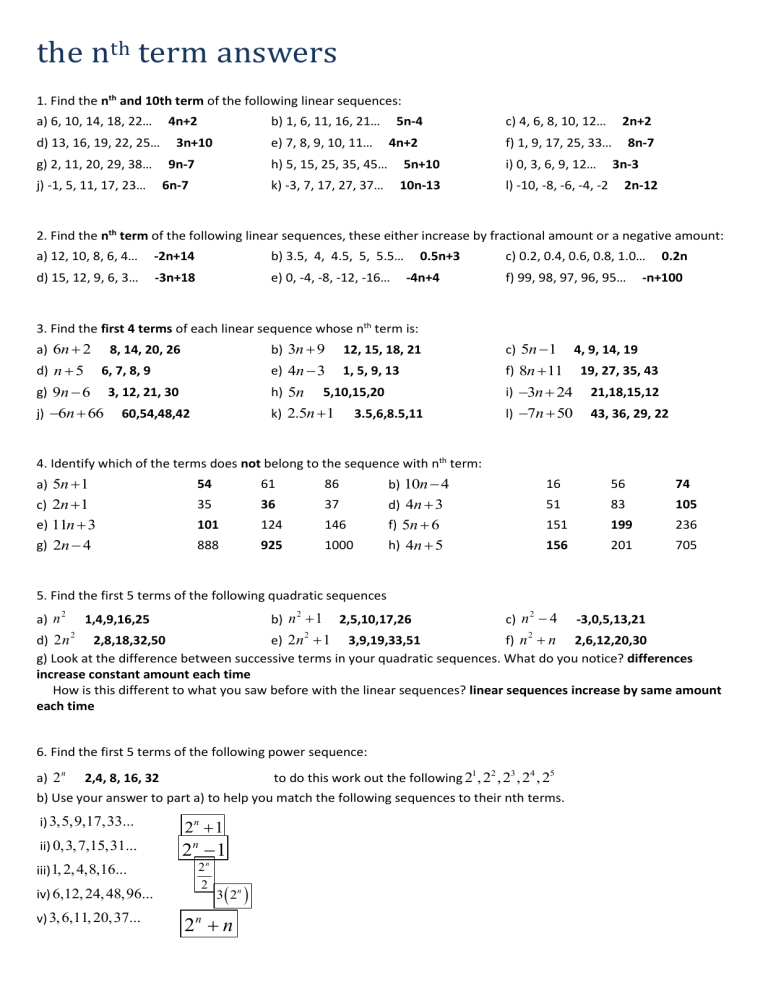



The Nth Term Answers




Y2vdaam I5gdlm
4n2 1, X1 n=1 n2 n!I don't get how the answer is #1/12#Answers Click here to see ALL problems on Proofs Question 1^23^2 (2n1)^2=1/3n (4n^21) Found 2 solutions by ikleyn, math_helper Answer by ikleyn () ( Show Source ) You can put this solution on YOUR website!



Performance Evaluation Ppt Download



Http Users Math Msu Edu Users Lawrence 3 Hmwk3 Solns Pdf
View this answer To solve the quadratic equation 2(n−1)(2n−1) (3n−1)(n1) =21 7n2−4n−= 0 2 ( n − 1) ( 2 n − 1) ( 3 n − 1) ( n 1) = 21 7 n 2 − 4 n − = 0 By First, it should be clear that the the limit of your original rational function is 2/3 you can see that by, as was originally suggested, dividing both numerator and denominator by n 2 (n^2 n 1) / (3n^2 1) equals (1 1/n 1/n 2)/(3 1/n 2) Obviously as n goes to infinity, each of those fractions with n in the denominator goes to 0 and so Prove by the principle of mathematical induction 7^2n 2^3n – 3 3n – 1 is divisible by 25 for all n ϵ N asked in Mathematical Induction by Prerna01 ( 5k points) mathematical induction



Http Www Webassign Net Resources Rogacalcet2 Testing Series Et Pdf




Answered 2 A 3 3n 1 C E 1 4n N 1 B Bartleby
Transcript Prove 1 2 3 n = (n(n1))/2 for n, n is a natural number Step 1 Let P(n) (the given statement)\ Let P(n) 1 2 3 n = (n(n1))/2 StepSigma(n=1, infinity) 2/(n^2 4n 3)Determine whether the series is convergent or divergent If it is convergent, find its sum 2^3n – 1 is divisible by 7, for all natural numbers n asked in Mathematics by AsutoshSahni ( 526k points) principle of mathematical induction



What Is The Value Of Lim N Infinity N N N 3n 3 4n 2 1 Quora



Solve 3n 2 4n 1 0 Youtube



3 Principle Of Mathematical Induction Prove Proof 1 5 9 4n 3 N 2n 1 Youtube




Given 2nh 3 G 3n 2o G 4n 2 G 3h 2o L Dh 1010 Clutch Prep
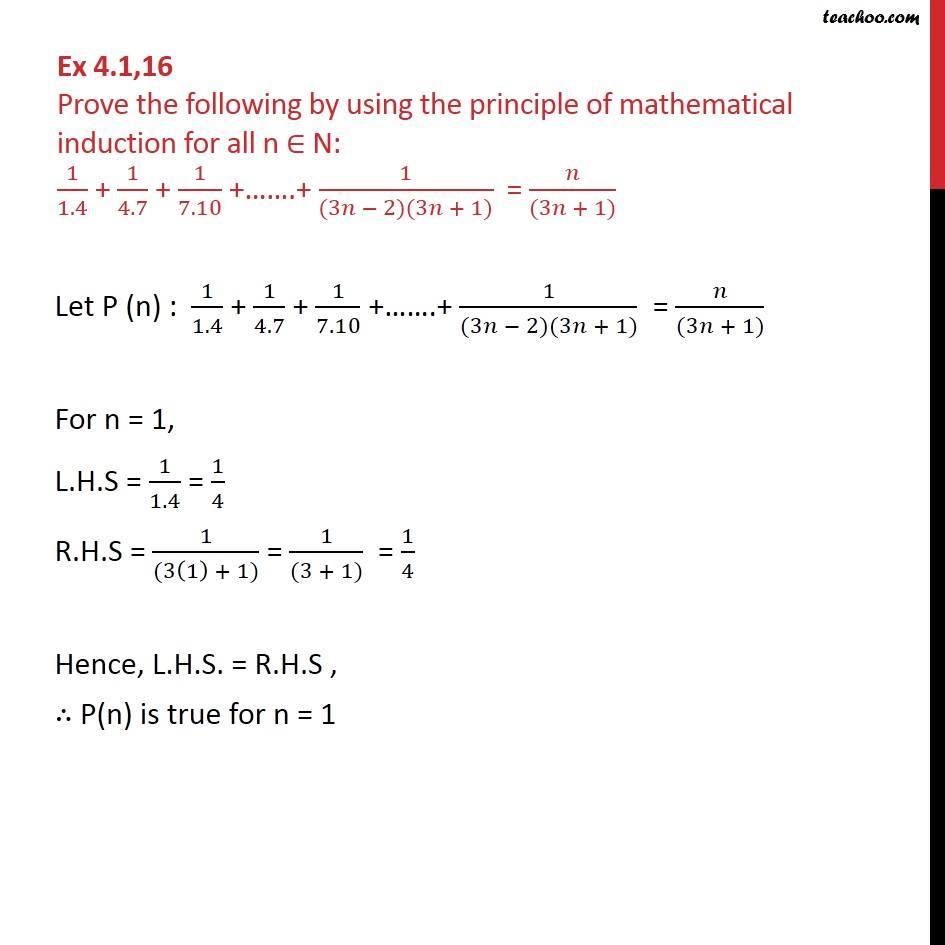



Ex 4 1 16 Prove 1 1 4 1 4 7 1 3n 2 3n 1 N 3n 1



Http Www Westmschools Org Userfiles 123 Classes 681 Userfiles 123 My files Function review Pdf Id




Let F And G Be Function From The Positive Integers To The Positive Integers Defined By The Equations F N 2n 1 G N 3n 1 Find The Compositions F Math Circ Math F G Math Circ Math G F Math Circ Math G And G Math Circ Math F Homework




Proof That 22 7 Exceeds P Wikipedia




Solve 2 N 1 4n 2 3n 1 Brainly In




Math 432 Hw 2 5 Solutions Pdf Free Download




10 Multiple Choice Questions Ppt Download




View Question The Ratio Of The Sum Of N Terms Of 2 Aps Is 3n 1 4n 3 Find The Ratio Of Their Mth Terms




Solve 2 N 1 4n 2 3n 1 Brainly In




To N Terms Dfracn 4n2 See How To Solve It At Qanda



Www2 Kenyon Edu Depts Math Kalaycioglu Calculus b Testsforconvergencewithanswers Pdf



Sequences



Http Www Math Usm Edu Lee Mat169 Exam1sol Pdf




Lim N Gtoo 1 2 3 4 5 6 2n Sqrt N 2 1 Sqrt 4n 2 1 1 1 3 2




Sum Tan 1 4n N 4 2n 2 2 Is Equal To Youtube
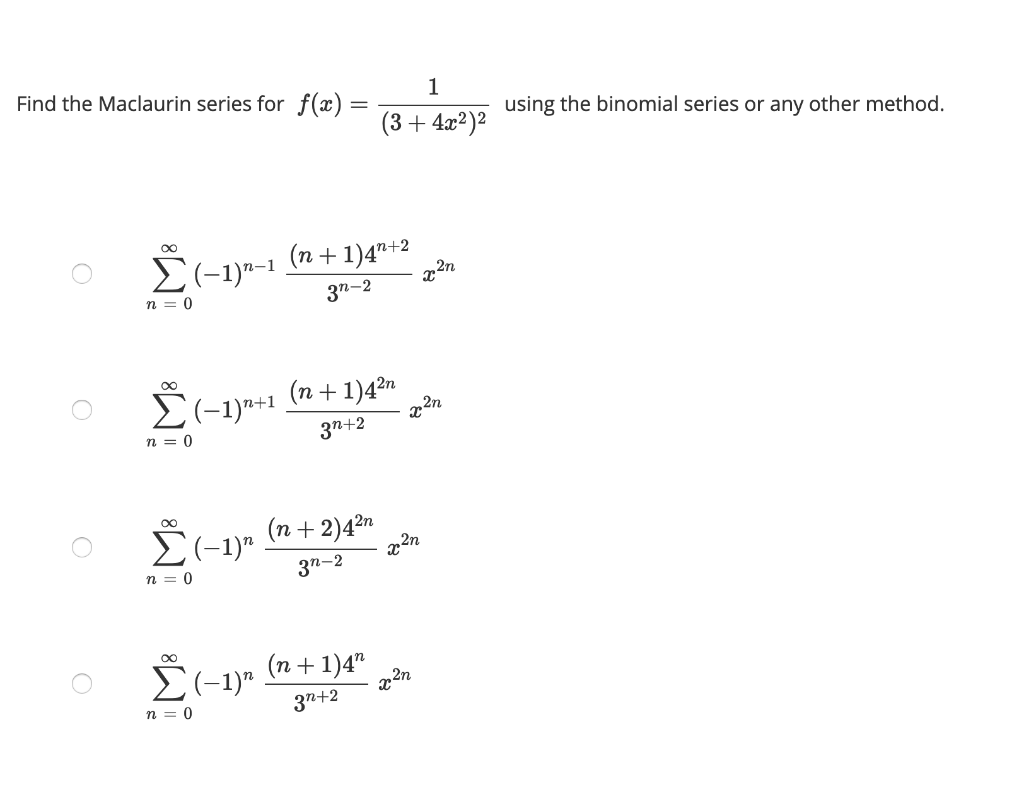



Find The Maclaurin Series For F X Using The Binomial Chegg Com



Limn 1 4n 2 1 1 4n 2 4 1 4n 2 9 1 3n 2 Sarthaks Econnect Largest Online Education Community



2



Http Www Math Colostate Edu Clayton Teaching M115f09 Exams Exam1solutions Pdf



Http Www Se Cuhk Edu Hk Manchoso 21 Estr04 Hw1 Sol Pdf



Www Mtholyoke Edu Courses Jmorrow Calculus Ii Factexsolns Pdf



1



Web Northeastern Edu Suciu Math3150 Math3150 Fa15 Hmw6 Solutions Pdf
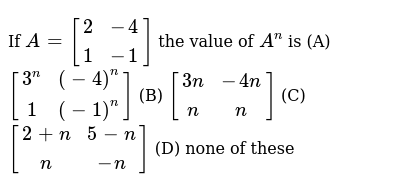



2 N 1 4n 2 3n 1




Performance Evaluation Ppt Download



Http Www Westerville K12 Oh Us Userfiles 46 Classes Solving linear equations variables on both sides answers Pdf Id
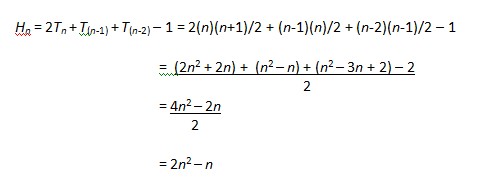



Problem To Ponder September 15 11 National Council Of Teachers Of Mathematics
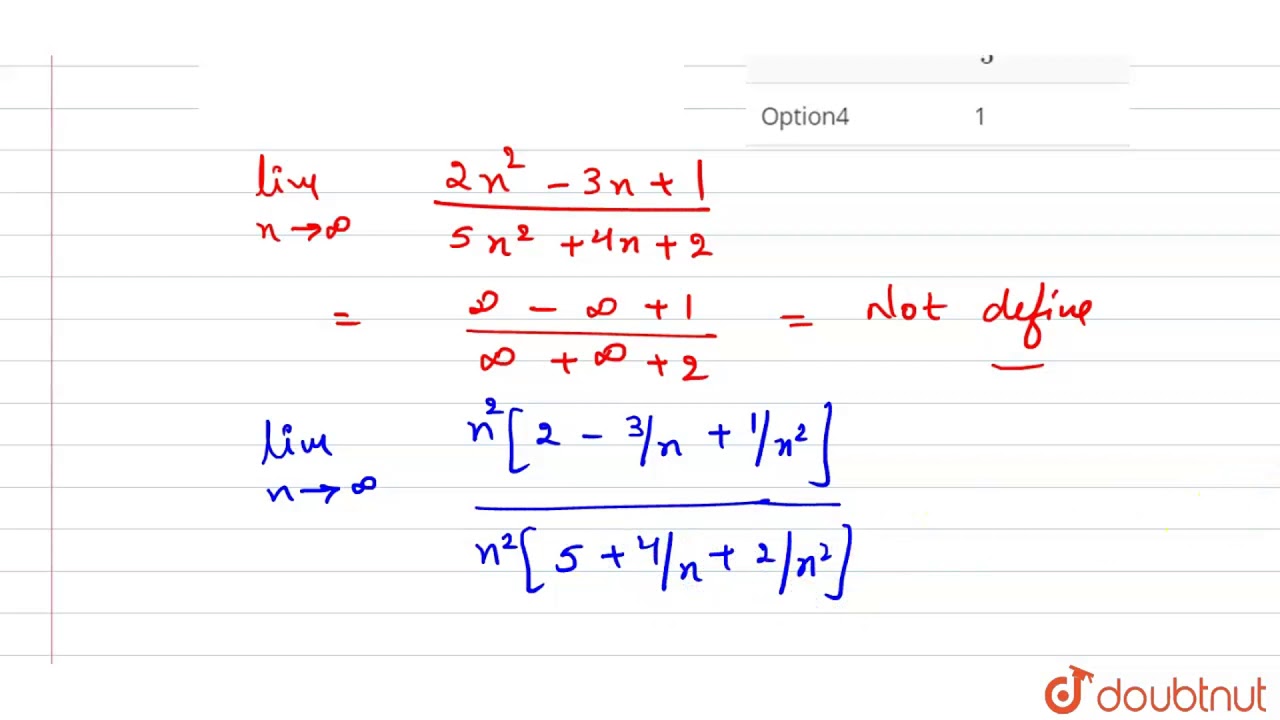



The Value Of Underset N To Oo Lim 2n 2 3n 1 5n 2 4n 2 Equals Youtube




1 Solution Select A Theta Notation From For Each Expression 1 F N




11 And Fourth Write Up Let M N2n 1 4n 1 N 2 Homeworklib




Tinh Lim Căn 4n 2 1 2n 1 Căn N 2 4n 1 N Nguyễn Anh



How To Prove 1 2 3 2 5 2 2n 1 2 N 4n 2 1 3 By Mathematical Induction Quora




22n 4 Their Interior Angles Are X 90 And Since The Ratio Of The Interior Angles Is 4 3 22n 4 90 X 90 22n 4 4 14n 44 22n 43 22n 23 3n



2



How Is This Proved By Mathematical Induction 1 2 2 2 3 2 2n 2 N 2n 1 4n 1 3 For The First 2n Positive Integers Quora



How Can You Prove That 1 5 9 Cdots 4n 3 2n 2 N Without Using Induction Mathematics Stack Exchange



2



Www Obhs Co Uk Assets Uploads Y9 Maths Hk Spring 2 The Nth Term Answers Pdf




Lim N Oo Sqrt N 3 2n 2 1 3sqrt N 4 1 3 4sqrt N 6 6n 5 2



Http Www D Umn Edu Jgreene Combinatorics Fall 15 Exam 2 Sol Pdf




Prove That 2n 1 2n 3 Dots 4n 1 3n 2 By Induction Mathematics Stack Exchange
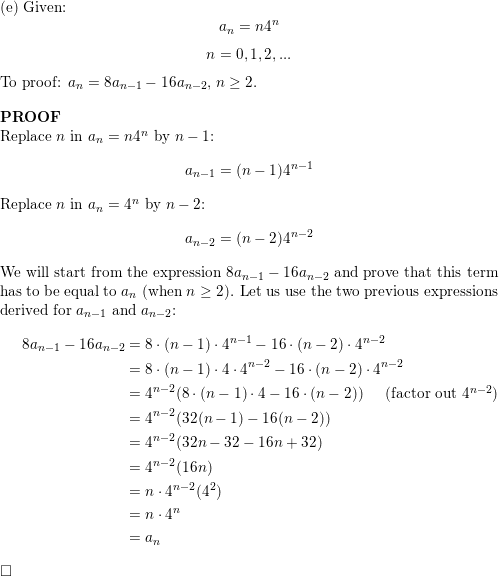



Is The Sequence A Math Square Math A Solution Of The Recurrence Relation Math A N 8a N 1 16a N 2 Math If A Math A N 0 Math B Math A N 1 Math C Math A N 2 N Math D Math A N 4 N Math



If Tn 2n 2 3n Then Prove That Sn N N 1 4n 11 6




N N 1 N 2 2n 2n 1 2n 2 3n 2 Redundancy Explained Bmc Software Blogs




4n 2 Or 2n 1 2n 1 Quantitative Comparison Questions



Translating Words To Math Bingo Card
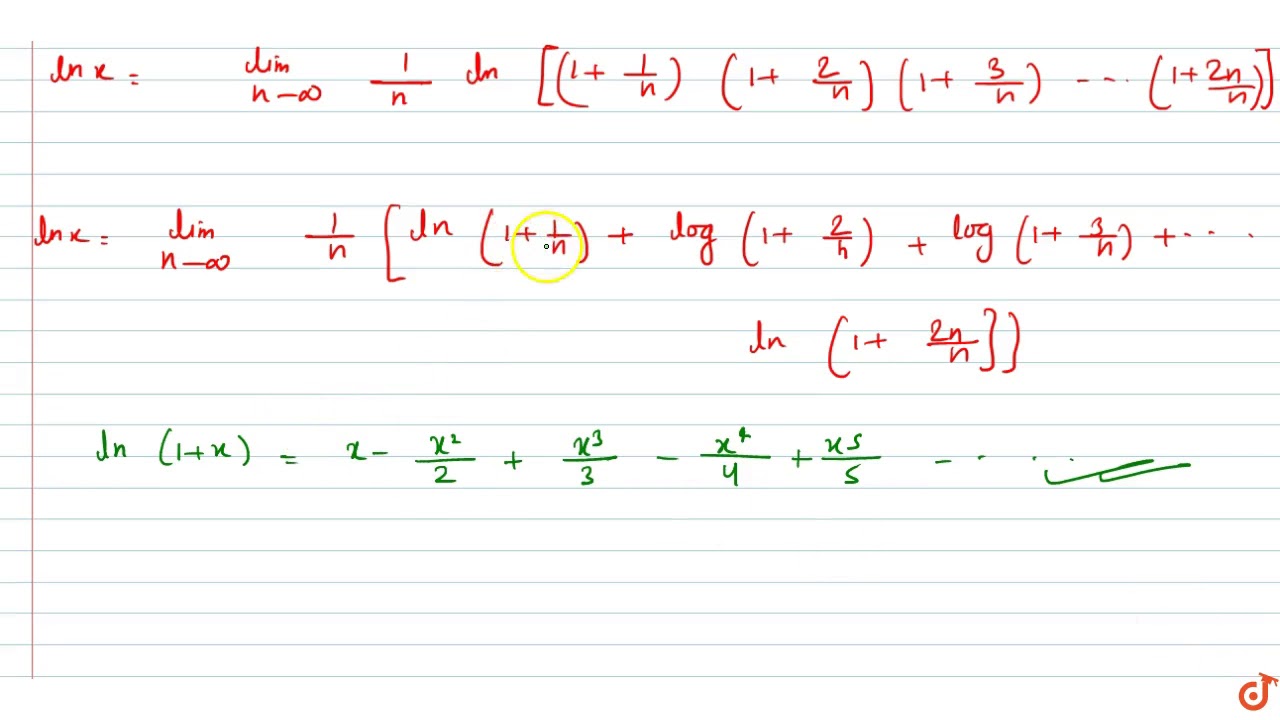



Lim N Gtoo N 1 N 2 3n N 2n 1 N Is Equal To Youtube



Solved If The Nth Partial Sum Sn Of An Infinite Series O 1 4n 3 0o An 4 3n An N 1 O 2 N 3 An 4 Is Given By 3n 1 N Sn 4 3n 1 3 2n Course Hero



Www Ualberta Ca Rjia Math214 Hwks Sol1 Pdf




The Value Of Lim N Oo Sqrt 3n 2 1 Sqrt 2n 2 1 4n 3 Is



1




Infinitely Many Solutions See How To Solve It At Qanda



Www3 Nd Edu Apilking Math Work Old exams Exams s14 Exam 3s14 solutions copy Pdf
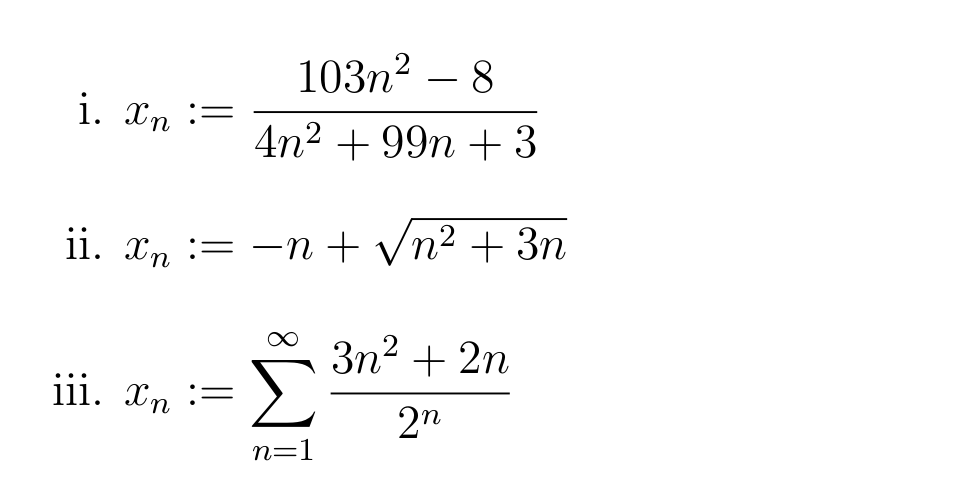



Answered 103n2 8 1 4n2 99n 3 1i Bartleby




Ncert Solutions For Class 9 10 11 And 12 Prove The Following By Using The Principle Of Mathematical Induction For All N N



2




Rd Sharma Solutions For Class 11 Maths Updated 21 22 Chapter 12 Mathematical Induction Download Free Pdf
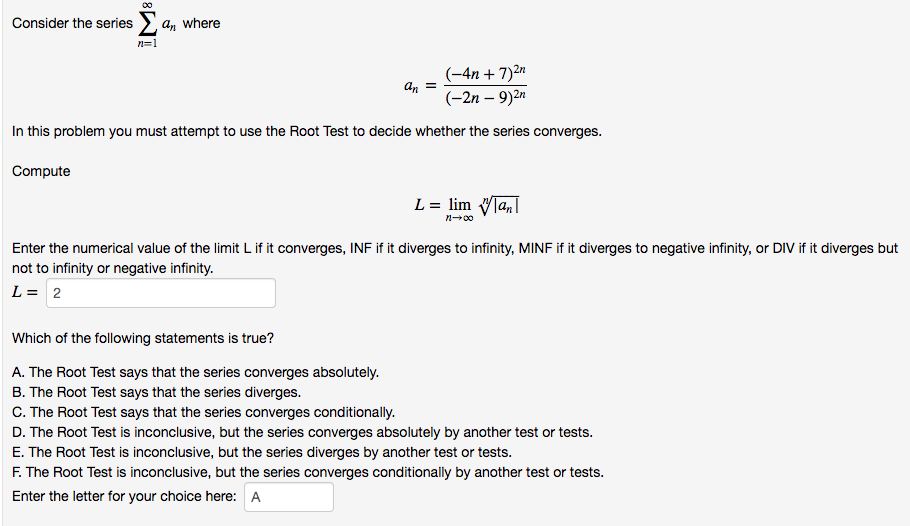



Answered Consider The Series An Where N 1 4n Bartleby




Data Structure And Algorithm Notes




1 Lim 4n 2 N 1 3 2n 2 2 Lim 2n 3n 3 1 N 3 N 2 3 Lim 3n 3 5n 1 N 2 4 4 Lim 2 3n 3 N 1 2 1 4n 5 5 Lim 2n



Proof Of Finite Arithmetic Series Formula By Induction Video Khan Academy




Discrete Math Proving 3n 8 4n 3 2n 1 Is O N 2 Using Definition Learnmath
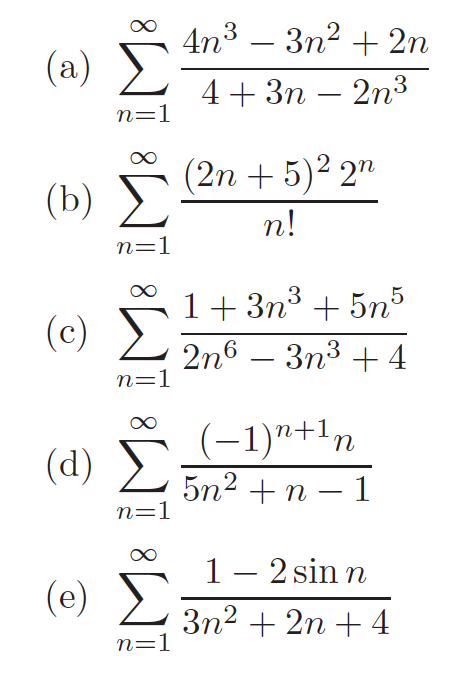



Determine Which Of The Following Series Are Chegg Com



Let Da A 1 N 6 A 1 2 2n 2 4n 2 A 1 3 3n 3 3n 2 3n Show That Da C For A 1 N Constant Sarthaks Econnect Largest Online Education Community



Solution Perform The Operations And Simplify 3n 2 5n 2 12n 2 13n 3 N 2 3n 2 4n 2 5n 6



Http Www Iitg Ac In Psm Indexing Ma353 Y09 Lecturenotema515aug3 Pdf
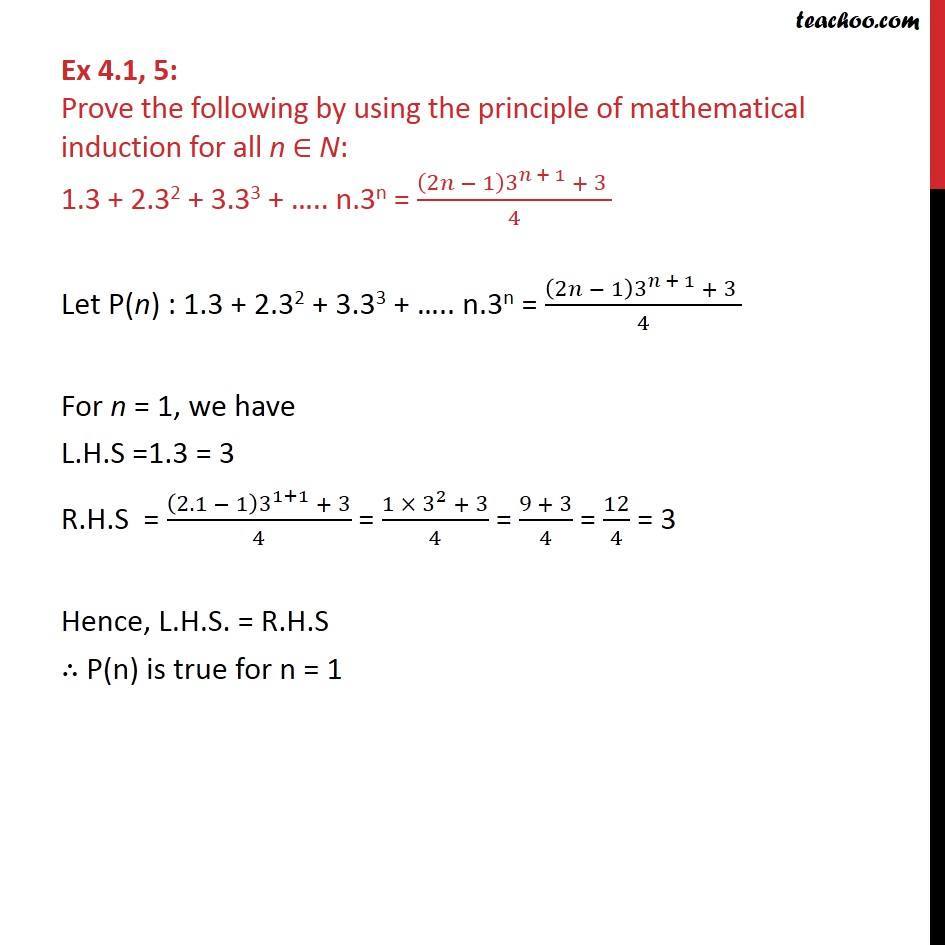



Ex 4 1 5 Prove 1 3 2 32 3 33 N 3n 2n 1 3n 1




Show That 4 3m 3 4n 2 2mn 16 9m2 9 16n2 Mathematics Topperlearning Com C8y0xpgg



How To Prove That Math 1 3 3 3 5 3 2n 1 3 2n 4 N 2 Math Using Mathematical Induction Quora
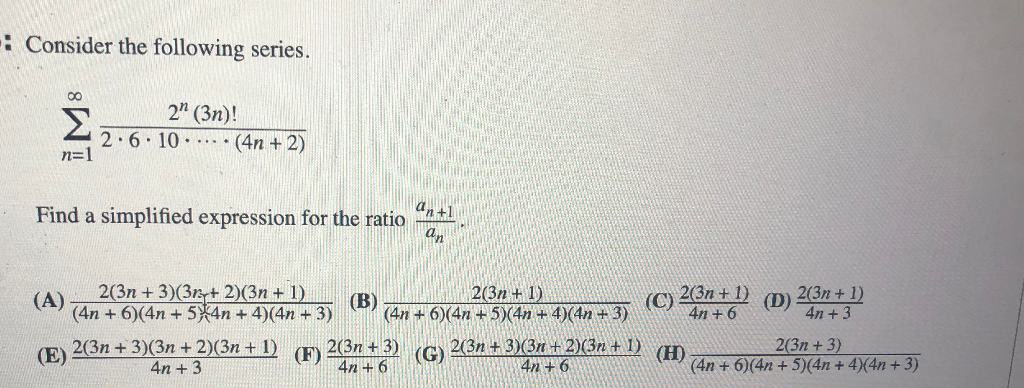



Consider The Following Series 21 3n Chegg Com



0 件のコメント:
コメントを投稿